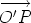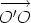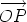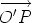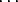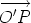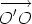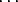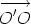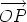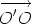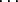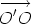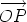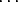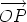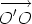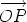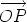|
||||||||||||||||||||||||||||||||||||||||||||||
|
14. Line Vectors
|
||||||||||||||||||||||||||||||||||||||||||||||
|
|
||||||||||||||||||||||||||||||||||||||||||||||
|
A collection of line vectors ( The resultant of this system is the vector R that is the sum of all the individual vectors. That is, 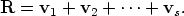
Suppose that O and O' are points. Let us compare the sum of the moments of the system of line vectors about the point O and about the point O'. For each line
We say that two systems of line vectors ( The calculation just carried out shows that this definition does not depend on the point O. When we consider the behaviour of a rigid body under the action of a system of forces, equivalent systems of forces produce the same effect on the body: the same resultant “push” or “pull” and the same amount of “twist”.
|
|||||||||||||||||||||||||||||||||||||||||||||
| Feedback |
|
|||||||||||||||||||||||||||||||||||||||||||||
© 2002-09 The University of Sydney. Last updated: 09 November 2009
ABN: 15 211 513 464. CRICOS number: 00026A. Phone: +61 2 9351 2222.
Authorised by: Head, School of Mathematics and Statistics.
Contact the University | Disclaimer | Privacy | Accessibility