 |
||||||||||||||||||||||||||||||||||||||
|
5. Division of a line segment
|
||||||||||||||||||||||||||||||||||||||
|
|
||||||||||||||||||||||||||||||||||||||
|
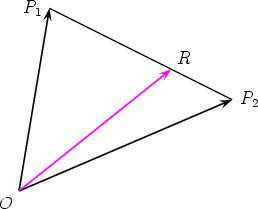
With an assigned point O as origin, the position of
any point P is given uniquely by the vector Let P1 and P2 be any points, and let R be a point on the line P1P2 such that R
divides the line segment P1P2 in the ratio m : n. That is, R is the point such that
As
which rearranges to give 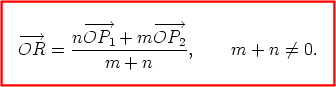
When m and n are both positive, the vectors As a special case of the general formula (1) we can obtain a formula for the position
vector In this case, m : n = 1 : 1 and so 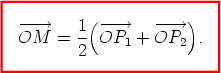
|
|||||||||||||||||||||||||||||||||||||
| Feedback |
|
|||||||||||||||||||||||||||||||||||||
© 2002-09 The University of Sydney. Last updated: 09 November 2009
ABN: 15 211 513 464. CRICOS number: 00026A. Phone: +61 2 9351 2222.
Authorised by: Head, School of Mathematics and Statistics.
Contact the University | Disclaimer | Privacy | Accessibility
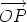 , which is called the
, which is called the 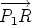
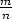
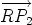 . Our task is to find the position vector of
. Our task is to find the position vector of 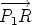
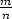
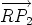 , we have
, we have 
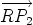 and therefore
and therefore
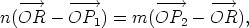
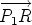 and
and 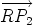 have the same direction,
since
have the same direction,
since 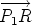
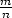
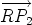 . This corresponds to the situation where
. This corresponds to the situation where 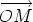 of the midpoint
of the midpoint